3.3.1: Some Examples of Multiple Quantification
- Page ID
- 1814
All of the following are sentences of predicate logic:
(1) (Vx)(Vy)Lxy
(2) (Ǝx)(Ǝy)Lxy
(3) (Ǝx)(Vy)Lxy
(4) (Ǝx)(Vy)Lyx
(5) (Vx)(Ǝy)Lxy
(6) (Vx)(Ǝy)Lyx
Let's suppose that 'L' stands for the relation of loving. What do these sentences mean?
Sentence (1) says that everybody loves everybody (including themselves). (2) says that somebody loves somebody. (The somebody can be oneself or someone else.) Sentences (3) to (6) are a little more tricky. (3) says that there is one person who is such that he or she loves everyone. (There is one person who is such that, for all persons, the first loves the second-think of God as an example.) We get (4) from (3) by reversing the order of the 'x' and 'y' as arguments of 'L'. As a result, (4) says that there is one person who is loved by everyone. Notice what a big difference the order of the 'x' and 'y' makes.
Next, (5) says that everyone loves someone: Every person is such that there is one person such that the first loves the second. In a world in . which (5) is true, each person has an object of their affection. Finally we get (6) out of (5) by again reversing the order of 'x' and 'y'. As a result, (6) says that everyone is loved by someone or other. In a world in which (6) is true no one goes unloved. But (6) says something significantly weaker than (3). (3) say that there is one person who loves everyone. (6) says that each person gets loved, but Adam might be loved by one person, Eve by another, and so on.
Can we say still other things by further switching around the order of the quantifiers and arguments in sentences (3) to (6)? For example, switching the order of the quantifiers in (6) gives
(7) (Ǝy)(Vx)Lyx
Strictly speaking, (7) is a new sentence, but it does not say anything new because it is logically equivalent to (3). It is important to see why this is so:
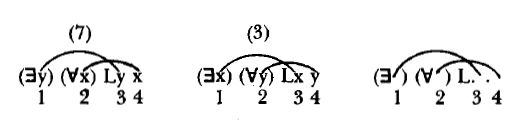
These diagrams will help you to see that (7) and (3) say exactly the same thing. The point is that there is nothing special about the variable 'x' or the variable 'y'. Either one can do the job of the other. What matters is the pattern of quantifiers and variables. These diagrams show that the pattern is the same. All that counts is that the variable marked at position 1 in the existential quantifier is tied to, or, in logicians' terminology, Binds the variable at position 3; and the variable at position 2 in the universal quantifier binds the variable at position 4. Indeed, we could do without the variables altogether and indicate what we want with the third diagram. This diagram gives the pattern of variable binding which (7) and (3) share.

